Click on the images to open a new tab and see them in full resolution.
3.3 Version 1 Answers
3.3 Version 2 Answers
3.3 Version 3 Answers
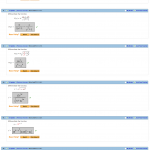
1. Differentiate the function. f(x) = log10(x^3 + 4)
2. Differentiate the function. f(x) = 9x ln(6x) – 9x
3. Differentiate the function. f(x) = sin(7 ln x)
4. Differentiate the function. f(x) = ln(121 sin^2x)
5. Differentiate the function. f(x) = sin(x) ln(3x)
6. Differentiate the function. f(x) = log2(xe^x)
7. Differentiate the function. f(x) = ln(x + (x^2 – 8)^1/2)
8. Differentiate the function. G(y) = ln (3y + 1)^2/sqrt(y^2 +1)
9. Differentiate the function. H’(z) = ln(sqrt(a^2 –z^2)/(a^2 + z^2))
10. Differentiate the function. y = sqrt(5 + 6e^5x)
11. Differentiate the function. y = 10^9-x^2
12. Differentiate the function. F(t) = 6^6tsin 2t
13. Differentiate the function. f(t) = tan(e^4t) + e^tan4t
14. Differentiate the function. y = [ln (1 + e^x)]^3
15. Find the derivative of the function. y = 5^8^x^2
16. Find an equation of the tangent line to the curve at the given point. Y = ln(x^2 – 2x + 1), (2,0)
17. Use logarithmic differentiation or an alternative method to find the derivative of the function.
Y = (x^3 + 2)^2 (x^5 + 4)^4
18. If f(x) = ln(4 + e^5x), find f’(0).
